Making errors with accuracy

Have you ever wondered whether you should use the words accurate, or precise? And if the data shows error, does this mean a mistake has been made?

The language of scientific inquiry is littered with terms that can be misused, misunderstood and incorrectly interchanged, especially when so many of these words are used in everyday language, but with slightly different meanings.

So, if you find yourself lacking confidence in using this vocabulary as a teacher, publisher, or editor, this blog post might be just what you need to end the uncertainty.

A little bit of history

During the past five or so centuries, scientists have made leaps and bounds in refining the scientific method. In this period, the scientific community has developed a specific vocabulary for presenting and talking about the measurements obtained from their investigations.

This vocabulary, however, was often not used consistently or correctly in secondary school science. There were differences in the terminology used between different exam boards, and thus differences in use between teaching materials from different publishers. A consensus on the correct terms and their meaning was needed.

In 2010, The Association for Science Education (ASE), in partnership with Nuffield Foundation, produced a booklet to ensure that educators, exam boards and publishers in the UK, were using terminology for scientific measurements, consistent with that used by scientists. This booklet, The Language of Measurement: Terminology used in school science investigations (ISBN 978 0 86357 424 5), is available on the internet and is an essential resource to any publisher or editor of secondary school science resources.

Accurate vs. Precise: Get the terminology exactly right

Let’s first look at the words ‘accurate’ and ‘precise’. At first glance, they may seem synonymous, especially given how interchangeable they are in everyday language:

“The brief gave an accurate summary of work involved in the copyedit.”

“The brief precisely stated what was required of the copyedit.”

However, The Language of Measurement defines the term ‘accuracy’ for use in school science as follows:

Accuracy: a measurement result is considered accurate if it is judged to be close to the true value. A quality denoting the closeness of agreement between a measured value and the true value of a measurand.

First of all, let’s pick up on the word ‘measurand’ – not one that gets thrown around very often! It is a noun, meaning a quantity to be measured.

Secondly, what is meant by ‘true value’? This is the value that would be obtained in an ideal measurement, completely free of errors.

So, ‘accuracy’, in scientific measurement, refers to how close a measured value is to the true value.

Precision, on the other hand, is defined as follows:

Precision: a quality denoting the closeness of agreement between (consistency, low variability of) measured values obtained by repeated measurements.

So precision, unlike accuracy, has nothing to do with the true value at all. It gives no indication of how close results are to the true value. A measurement is considered precise if values are closely grouped.

Precision can be defined mathematically by calculating, for example, the standard deviation in a set of values.

Precision and accuracy are often presented to students using the darts or archery analogy:

‍

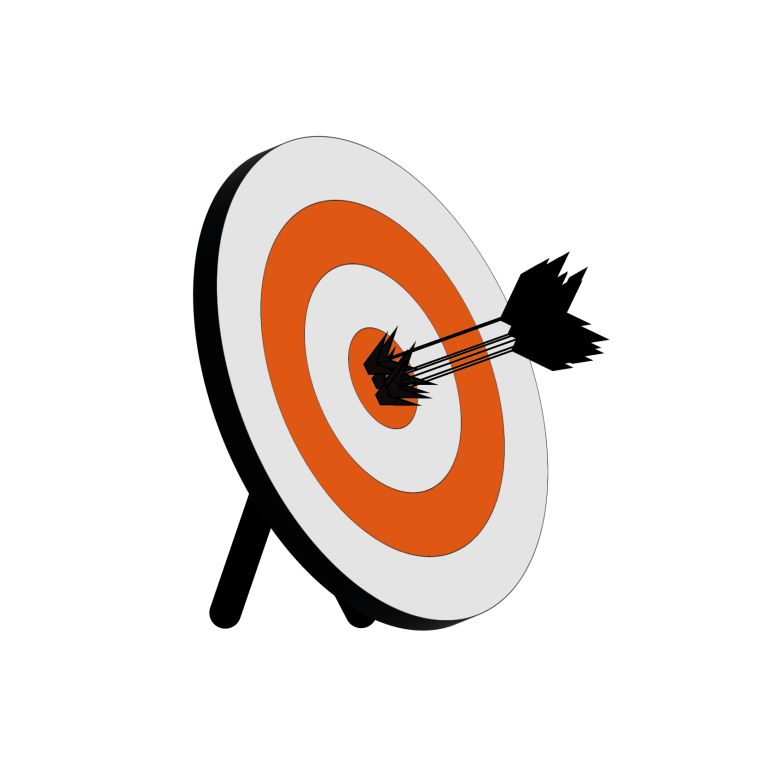
Don’t make a mistake with error

So, accuracy and precision are two different ways of describing of observational (measured) error.

But what do we actually mean by ‘error’? In science, this does not necessarily mean a mistake, which is an, umm, error that students often make!

Error in scientific inquiry, or more accurately measurement error, refers to variability, and is an inevitable part of measurement. It can be caused by the instruments used, the way they are used, or other factors beyond our control.

The definition given in The Language of Measurement is:

Measurement error: the difference between a measured value and the true value of a physical quantity being measured, where a true value is thought to exist.

Scientists try to correct for known errors, for example by applying corrections from instrument calibrations, but any error whose value is unknown is a source of uncertainty in the data.

Measurement errors can include components of systematic and/or random error. These are defined in The Language of Measurement as:

Systematic error: component of measurement error due to measurement results differing from the true value by a consistent amount each time a measurement is made.

Random error: component of measurement error due to measurement results varying in an unpredictable way from one measurement to the next.

Ultimately, systematic error will affect accuracy, while random error will reduce precision. Looking back at our archery analogy:

Systematic error

• Systematic errors can be due to the environment, methods of observation, or instruments used. For example, in the case of this archery round, it might be that the sight on the archer’s bow was pointing slightly to the left.
• Systematic error will have the same value or proportion for every measurement.
• Other examples of sources of systematic error include reading a meniscus consistently above or below eye level when measuring the volume of a liquid, or using a thermometer to measure temperature outside of its normal range.
• If the cause of systematic errors can be identified, then the effect can be reduced or eliminated. For example, by calibration of instruments.

Random error

• Random error is due to chance differences that are part of the measuring process.
• Random error will change unpredictably with each measurement. For example, in the case of this archery round, it might have been a gusty day with random bursts of wind in all directions.
• Other examples of sources of random error include posture changes between individuals when measuring height, or changes in temperature when measuring the volume of a gas.
• Variation due to random error can’t be corrected for, but the effect can be reduced by making repeated measurements and calculating the mean.

Why is precise use of language important?

It is well understood that specialist terminology allows people to communicate clearly and effectively. This is inherently important in the field of scientific research, where collaborations between scientists from different countries are common.

The UK national curriculum for key stage 4 science states that students must be able to ‘evaluate data in terms of accuracy, precision … and identify potential sources of random and systematic error.’

Therefore, in secondary science, it is important that students are introduced carefully to this vocabulary. They must be guided to separate how they use such terms in everyday life, from the specific use of these terms in discussing data. The correct use of these terms is expected and rewarded by UK awarding bodies in examinations and is detailed in their specifications, following the standard set out by the ASE.

We hope you found this blog post useful, and that you will now be able to talk about and present observations in scientific inquiry accurately, using precise language that is free from error, with no more uncertainty!

In a future blog post, we will extend this topic by addressing the ‘other’ three Rs—reliability, repeatability, and reproducibility—but in the meantime we’d love to hear your thoughts on the topic, or any situations you’ve encountered where accurate (or is that precise?!) use of language came to save the day!

‍

‍